Задать вопрос Регистрация
Помочь другим

  • Помогите, пожалуйста, решить дифференциальное уравнение

    (x/(sqrt(x^2-y^2)-1))dx-(y/(sqrt(x^2-y^2)))dy=0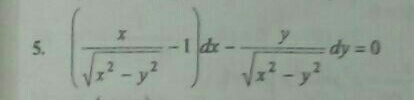

    question img

Ответы 2

  • Смотри полученное решение
    answer img
  • \Big (\frac{x}{\sqrt{x^2-y^2}}-1\Big )\, dx-\frac{y}{\sqrt{x^2-y^2}}\, dy=0\\\\\frac{x-\sqrt{x^2-y^2}}{\sqrt{x^2-y^2}}\, dx=\frac{y}{\sqrt{x^2-y^2}}\, dy\\\\\frac{dy}{dx}=\frac{(x-\sqrt{x^2-y^2})\cdot \sqrt{x^2-y^2}}{y\cdot \sqrt{x^2-y^2}}\, \Big |\frac{:x}{:x}\\\\y'=\frac{(1-\sqrt{1-(\frac{y}{x})^2}\, )\cdot \sqrt{1-(\frac{y}{x})^2}}{\frac{y}{x}\cdot \sqrt{1-(\frac{y}{x})^2}}\\\\t=\frac{y}{x}\; ,\; \; y'=t'x+t\\\\t'x+t=\frac{(1-\sqrt{1-t^2})\cdot \sqrt{1-t^2}}{t\cdot \sqrt{1-t^2}}=\frac{\sqrt{1-t^2}-(1-t^2)}{t\cdot \sqrt{1-t^2}}t'x+t=\frac{\sqrt{1-t^2}-1+t^2}{t\cdot \sqrt{1-t^2}}\\\\t'x=\frac{\sqrt{1-t^2}-1+t^2}{t\, \sqrt{1-t^2}}-t=\frac{\sqrt{1-t^2}}{t\, \sqrt{1-t^2}}-\frac{1-t^2}{t\, \sqrt{1-t^2}}-t\\\\t'x=\frac{1}{t}-\frac{\sqrt{1-t^2}}{t}-t\; \; \to \; \; t'=\frac{1}{x}\cdot \Big (\frac{1}{t}-\frac{\sqrt{1-t^2}}{t}-t\Big )\\\\\frac{dt}{dx}=\frac{1}{x}\cdot (\frac{1}{t}-\frac{\sqrt{1-t^2}}{t}-t)\\\\ \frac{dt}{\frac{1}{t}-\frac{\sqrt{1-t^2}}{t}-t}=\frac{dx}{x}\\\\\frac{t\, dt}{1-\sqrt{1-t^2}-t^2}=\frac{dx}{x}a)\; \int \frac{t\, dt}{(1-t^2)-\sqrt{1-t^2}}=[\, u=1-t^2\; ,\; du=-2t\, dt\, ]=-\frac{1}{2}\int \frac{du}{u-\sqrt{u}}=\\\\=[\, u=z^2\; ,\; du=2z\, dz\; ,\; z=\sqrt{u}\, ]=-\frac{1}{2}\int \frac{2z\, dz}{z^2-z}=\\\\=-\int \frac{z\, dz}{z(z-1)}=-\int \frac{dz}{z-1}=-ln|z-1|+lnC_1=ln\frac{C_1}{|z-1|}=\\\\=ln\frac{C_1}{|\sqrt{u}-1|}=ln\frac{C_1}{|\sqrt{1-t^2}-1|}=ln\frac{C_1}{|\sqrt{1-\frac{y^2}{x^2}}-1|}=ln\frac{C_1\, |x|}{|\sqrt{x^2-y^2}-x|}\; ;\\\\b)\; \; \int \frac{dx}{x}=ln|x|+ln C_2=ln(C_2|x|)\; ;ln\frac{C_1|x|}{|\sqrt{x^2-y^2}\, -x|}=ln(C_2|x|)\\\\\frac{C_1|x|}{|\sqrt{x^2-y^2}\, -x|}=C_2|x|\\\\\sqrt{x^2-y^2}\, -x=C\; \; \; \; (\, C= \frac{C_1}{C_2}\, )
    answer img

    • Автор:

      marin
    • 5 лет назад
    • 0

  • Добавить свой ответ

Еще вопросы

Топ пользователей

Войти через Google

 или

Забыли пароль?

У меня нет аккаунта, я хочу Зарегистрироваться

How much to ban the user?
1 hour 1 day 100 years