Задать вопрос Регистрация
Помочь другим

  • Помогите решить систему уравнений)Буду очень благодарна)
    Номер 535​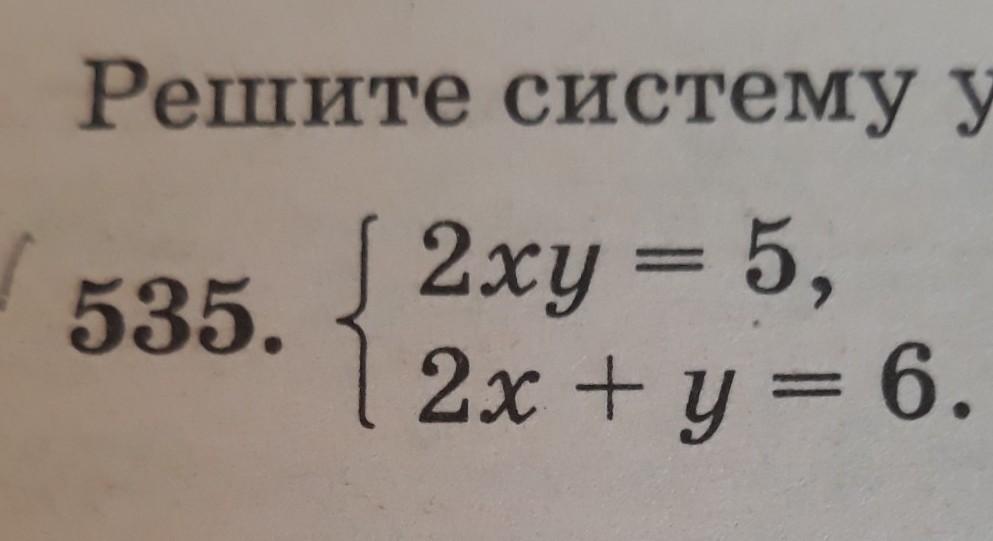

    question img

Ответы 4

  • Спасибо большое!)
  • Удачи.

  • Ответ: x₁=2,5    y₁=1      x₂=0,5       y₂=5.

    Объяснение:

    {2x+y=6     y=6-2x

    {2*xy=5      

    2*x*(6-2x)=5    

    12x-4x²=5      

    4x²-12x+5=0   D=64    √D=8

    x₁=2,5        ⇒     y₁=6-2*2,5=6-5=1

    x₂=0,5       ⇒      y₂=6-2*0,5=6-1=5.

    • Автор:

      erinbcbr
    • 5 лет назад
    • 0

  • \displaystyle\tt\left \{ {{2xy=5~~~} \atop {2x+y=6}} ight. \left \{ {{2xy=5~~~} \atop {2x=6-y}} ight. \left \{ {{(6-y)y=5} \atop {2x=6-y~~}} ight.

    (6 - y)y = 5

    6y - y² = 5

    -y² + 6y - 5 = 0 | : (-1)

    y² - 6y + 5 = 0

    D = 36 - 20 = 16 = 4²

    \displaystyle\tt y_1=\frac{6+4}{2} =5\\\\\\y_2=\frac{6-4}{2}=1

    \displaystyle\tt\left \{ {{2xy=5} \atop {y_1=5~~}} ight. \left \{ {{2x\cdot5=5} \atop {y_1=5~~~~}} ight. \left \{ {{10x=5} \atop {y_1=5~}} ight.\left \{ {{x_1=0.5} \atop {y_1=2~~}} ight.

    \displaystyle\tt\left \{ {{2xy=5} \atop {y_2=1~~}} ight. \left \{ {{2x=5} \atop {y_2=1}} ight. \left \{ {{x_2=2.5} \atop {y_2=1~~}} ight. \\\\\\\left \{ {{x_1=0.5;~x_2=2.5} \atop {y_1=2;~y_2=1~~~~}} ight.

    Ответ: x₁ = 0,5, y₁ = 2; x₂ = 2,5, y₂ = 1

  • Добавить свой ответ

Еще вопросы

Топ пользователей

Войти через Google

 или

Забыли пароль?

У меня нет аккаунта, я хочу Зарегистрироваться

How much to ban the user?
1 hour 1 day 100 years